Projection methods for Neural Field equations

Avitabile, D.

Abstract

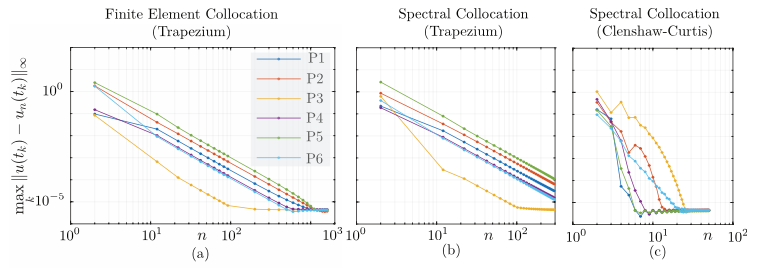
Neural field models are nonlinear integro-differential equations for the evolution of neuronal activity, and they are a prototypical large-scale, coarse-grained neuronal model in continuum cortices. Neural fields are often simulated heuristically and, in spite of their popularity in mathematical neuroscience, their numerical analysis is not yet fully established. We introduce generic projection methods for neural fields, and derive a-priori error bounds for these schemes. We extend an existing framework for stationary integral equations to the time-dependent case, which is relevant for neuroscience applications. We find that the convergence rate of a projection scheme for a neural field is determined to a great extent by the convergence rate of the projection operator. This abstract analysis, which unifies the treatment of collocation and Galerkin schemes, is carried out in operator form, without resorting to quadrature rules for the integral term, which are introduced only at a later stage, and whose choice is enslaved by the choice of the projector. Using an elementary timestepper as an example, we demonstrate that the error in a time stepper has two separate contributions: one from the projector, and one from the time discretisation. We give examples of concrete projection methods: two collocation schemes (piecewise-linear and spectral collocation) and two Galerkin schemes (finite elements and spectral Galerkin); for each of them we derive error bounds from the general theory, introduce several discrete variants, provide implementation details, and present reproducible convergence tests.

Reproduce the results of the paper, download or play with the  code in the code-capsule below

Links‌

BibTeX‌

@article{avitabile2023projection,
  title={Projection Methods for Neural Field Equations},
  author={Avitabile, Daniele},
  journal = {SIAM Journal on Numerical Analysis}, 
  volume = {61},
  number = {2},
  pages = {562-591},
  year = {2023},
  doi = {10.1137/21M1463768},
  eprint = {https://doi.org/10.1137/21M1463768}
}