Spot dynamics in a reaction-diffusion model of plant root hair initiation

Authors

Avitabile, D., Bren͂a, V.F. and Ward, M.J.

Abstract

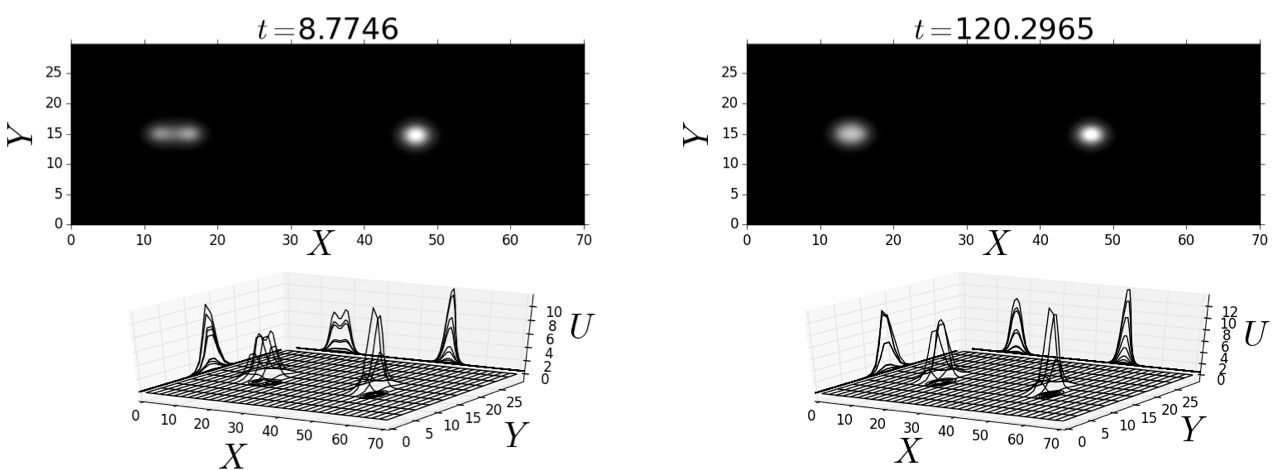
We study pattern formation in a 2D reaction-diffusion (RD) subcellular model characterizing the effect of a spatial gradient of a plant hormone distribution on a family of G-proteins associated with root hair (RH) initiation in the plant cell Arabidopsis thaliana. The activation of these G-proteins, known as the Rho of Plants (ROPs), by the plant hormone auxin is known to promote certain protuberances on RH cells, which are crucial for both anchorage and the uptake of nutrients from the soil. Our mathematical model for the activation of ROPs by the auxin gradient is an extension of the model of Payne and Grierson [PLoS ONE, 4 (2009), e8337] and consists of a two-component Schnakenberg-type RD system with spatially heterogeneous coefficients on a 2D domain. The nonlinear kinetics in this RD system model the nonlinear interactions between the active and inactive forms of ROPs. By using a singular perturbation analysis to study 2D localized spatial patterns of active ROPs, it is shown that the spatial variations in the nonlinear reaction kinetics, due to the auxin gradient, lead to a slow spatial alignment of the localized regions of active ROPs along the longitudinal midline of the plant cell. Numerical bifurcation analysis together with time-dependent numerical simulations of the RD system are used to illustrate both 2D localized patterns in the model and the spatial alignment of localized structures.

Links

BibTeX

@article{avitabile2018spot,
  title={Spot dynamics in a reaction-diffusion model of plant root hair initiation},
  author={Avitabile, Daniele and Bren͂a, Victor F and Ward, Michael J},
  journal={SIAM Journal on Applied Mathematics},
  volume={78},
  number={1},
  pages={291--319},
  year={2018},
  publisher={SIAM}
}